Jumps in granular flows down inclines

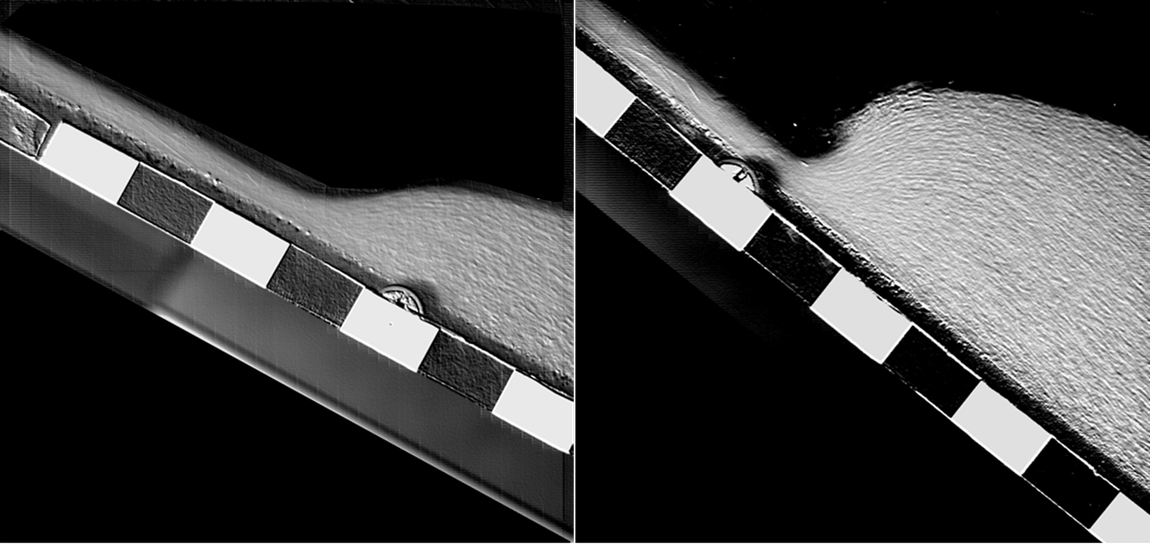
When a flow is obstructed by an obstacle or deflected by a sudden change in slope, jumps or waves can be formed. Such discontinuities are ubiquitous in many environmental situations involving various kinds of fluids: urban floods in road junctions, outlet dams, weirs and bridge piles in high slopes rivers, fish ways, impact of flows such as breaking water waves, snow avalanches, tsunami and debris flows against protection structures.

The project focuses on jumps occurring in granular materials, in particular those relevant for avalanches impacting structures.

Many studies have shown that traditional shallow-water shock equations do a good job in predicting granular jumps if the flow is rapid and relatively dense. In the case of granular flows down inclines however, very recent laboratory tests have shown the existence of behaviours that these equations fail to predict: transition from steep jumps with recirculation to frictional diffuse jumps, occurrence of a shock in density if the incident flow becomes dilute, and formation of dead zones for very diffuse jumps. Those transitions modify the size, shape and properties of the jumps and clearly show the limits of the traditional shock equations for diffuse frictional jumps and compressible jumps.

The project aims at studying these different granular jump patterns with the help of laboratory tests coupled with numerical simulation using DEM (discrete element method), in order to tackle all regimes, understand the internal processes at stake and develop new analytic solutions for each kind of jump.

The main expected breakthrough concerns the identification of a complete phase diagram for granular jump patterns depending on the Froude number, the thickness and the density of the incident flows, and the particle diameter relative to the typical basal roughness size as well as the size of the obstacle (for jumps formed around obstacles).

Beyond the contribution to some aspects of the rheology of granular flows yet to be solved, this work is of utmost importance for a better understanding of granular flows around obstacles and the design rules of avalanche protection dams that still rely on the classical equations.