Results published in 2014 in Granular Matter
By coupling experimental observations and mechanical modelling, the researchers from IRSTEA Grenoble have developed a new computational method to simplify the identification of individual snow grains in the complex network of ice crystals that makes up the snow.
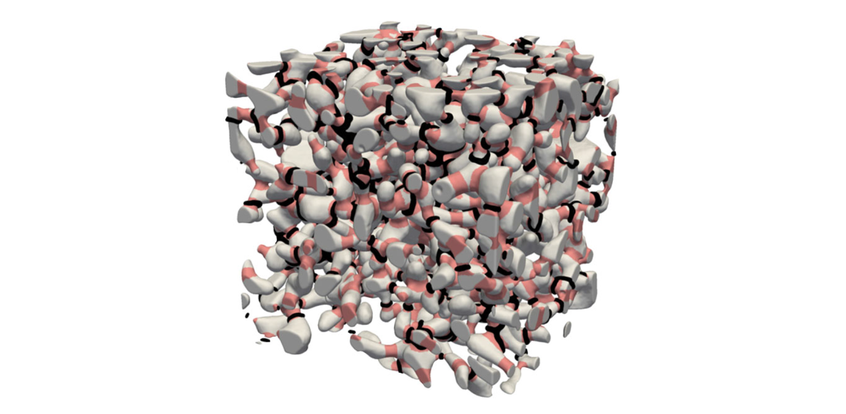
Snow is made of an assembly of sintered ice crystals forming a complex skeleton. At the microscale, the ice matrix has zones of mechanical weakness that delimit entities which can be viewed as snow grains. Rapid deformations of snow are mostly controlled by the dynamics of these mechanically-defined snow grains, their spatial rearrangements and contact interactions. When it comes to understanding the mechanical behaviour of snow in avalanche release processes for instance, the thorough identification of snow grains is of prime importance.
Geometrical clues of weakness
By comparing the distribution of mechanical stresses in snow samples and their 3D architecture, the researchers from IRSTEA have developed a new algorithm which uses local geometrical clues to infer zones of mechanical weakness in the ice matrix, and therefore simplifies the segmentation of the snow microstructure into grains.
When compared to the segmentation method based on the full computation of mechanical stress in the matrix, their algorithm enables a drastic reduction of the computational costs by a factor of 20. These results pave the way to a better consideration of the granular nature of snow in numerical simulations, in particular during rapid deformation phenomena where the large rearrangements of the microstructure render direct simulations extremely time consuming.
Figure caption
3D view of a snow sample (1 cm3). The bonds between snow grains were defined using either the method of mechanical stress computation (black rings) or the new algorithm based on geometrical clues (pink zones).
Reference
P. Hagenmuller, G. Chambon, F. Flin, S. Morin and M. Naaim, 2014: Snow as a granular material: assessment of a new grain segmentation algorithm. Granular Matter 16: 421-432.



